A Control Method for Integrating Hybrid Power Source into an Islanded Microgrid through CHB Multilevel Inverter

ABSTRACT:

This paper proposes a control strategy for an islanded microgrid to effectively coordinate hybrid power source (HPS) units and to robustly control individual interfaced inverters under unbalanced and nonlinear load conditions. Cascaded H-bridge (CHB) multilevel inverters are flexibly deployed in order to enhance the power quality and redundancy. The HPS employs fuel cell (FC) as the main and super capacitors (SC) as complementary power sources. Fast transient response; high performance; and high power density are the main characteristics of the proposed HPS system. The presented control strategy consists of a power management strategy for the HPS units and a voltage control strategy for the CHB multilevel inverter. A multi proportional resonant (multi-PR) controller is employed to regulate the load voltage at unbalanced and nonlinear load conditions. The proposed multi-PR controller includes a fundamental voltage controller with harmonic compensators. Digital time domain simulation studies in the PSCADIEMTDC environment are given to verify the overall proposed system performance.

KEYWORDS:    

                                                                                                  

1.      Hybrid power source

2.       Fuel cell

3.       Supercapacitor

4.      CHB multilevel inverter

5.       Multi-PR

SOFTWARE: MATLAB/SIMULINK

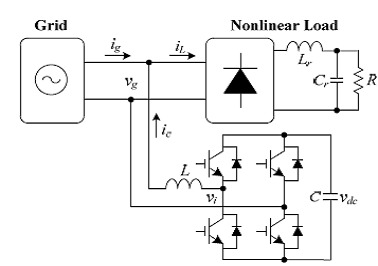
CIRCUIT DIAGRAM:

Fig. I. Proposed structure of the hybrid FC/SC power source.

CONTROL SYSTEM

Fig. 2. Proposed control strategy of hybrid FC/SC power source

EXPECTED SIMULATION RESULTS:

Fig. 3. Microgrid response to unbalanced and nonlinear load changes;

(a) Instantaneous real and reactive power. (b) Positive-sequence, negativesequence,

and harmonic components of load

Fig. 4. (a) Instantaneous current waveforms, (b) switching patterns of the

output voltage, and (c) voltage waveforms of each phase of the DG unit's

CHB inverter due to the nonlinear load connection.

Fig. 5. (a) Instantaneous current waveforms, (b) switching patterns of the

output voltage, and (c) voltage waveforms of each phase of the DG unit's

CHB inverter due to the single-phase load disconnection.

Fig. 6. (a) voltage THD, and (b) voltage unbalance factor at DG unit

terminal.

Fig. 7. The dc-link voltage waveforms to the unbalanced and nonlinear

load changes.

  

Fig. 8. Dynamic response of the DG unit to load changes: currents of FC

stacks and SC units of each HPS, (a) phase a, (b) phase b, and (c) phase c.

 CONCLUSION:

This paper presents an effective control strategy for an autonomous microgrid considering the HPS and CHB multilevel inverter under unbalanced and nonlinear load conditions. The proposed strategy includes power management of the hybrid FC/SC power source and the CHB multilevel inverter voltage control. The main characteristics of the proposed HPS are high performance; high power density; fast transient response. Furthermore, a multi-PR controller is presented to regulate the voltage of the CHB multilevel inverter in the presence of unbalanced and nonlinear loads. The performance of the proposed control strategy is investigated using PSCADIEMTDC software. The results show that the proposed strategy:

• robustly regulates the voltage of the microgrid under unbalanced and nonlinear load conditions;

• reduces THD and improves power quality by using CHB multilevel inverters;

• enhances the dynamic response of the microgrid;

• accurately balances the dc-link voltage of each H-bridge cell; and

• effectively manages the power among the power sources in the HPS system.

 REFERENCES:

[l] W. Liu, J. F. Chen, T. Liang, and R. Lin, "Multicascoded sources for a high-efficiency fuel-ceU hybrid power system in high-voltage application," IEEE Trans. Power Electron., vol. 26, pp. 931-942, Mar. 2011.

[2] IEEE Recommended Practice for Electric Power Distribution for Industrial Plants. ANSIIIEEE Std. 141, 1993.

[3] IEEE Recommended Practices and Requirements for Harmonic Control in Electrical Power System. IEEE Std. 519, 1992.

[4] J. Pereda and J. Dixon, "23-level inverter for electric vehicles using a single battery pack and series active filters," IEEE Trans. Veh. Techno!., vol. 61, pp. 1043-1051, Mar. 2012.

[5] A. Ghazanfari, M. Hamzeh, H. Mokhtari, and H. Karimi, "Active power management of multihybrid fuel celIlsupercapacitor power conversion system in a medium voltage microgrid," IEEE Trans. Smart Grid, vol. 3, pp. 1903-1910, Dec. 2012.

Frequency Adaptive Fractional Order Repetitive Control of Shunt Active Power Filters

ABSTRACT:

Repetitive control which can achieve zero steady-state error tracking of any periodic signal with known integer period, offers active power filters a promising accurate current control scheme to compensate the harmonic distortion caused by nonlinear loads. However, classical repetitive control cannot exactly compensate periodic signals of variable frequency, and would lead to significant performance degradation of active power filters. In this paper a fractional order repetitive control strategy at fixed sampling rate is proposed to deal with any periodic signal of variable frequency, where a Lagrange interpolation based fractional delay filter is used to approximate the factional delay items. The synthesis and analysis of fractional-order repetitive control systems are also presented. The proposed fractional-order repetitive control offers fast on-line tuning of the fractional delay and the fast update of the coefficients, and then provides active power filters with a simple but very accurate real-time frequency adaptive control solution to the elimination of harmonic distortions under grid frequency variations. A case study of single-phase shunt active power filter is conducted. Experimental results are provided to demonstrate the validity of the proposed fractional-order repetitive control.

KEYWORDS:

1.      Active power filter

2.      Fractional order

3.       Repetitive control

4.      Frequency variation

SOFTWARE: MATLAB/SIMULINK

CIRCUIT DIAGRAM:

Fig. 1. Single-phase shunt APF connected to the grid with nonlinear load.

CONTROL SYSTEM

Fig. 2. Dual-loop control scheme for single-phase APF.

  EXPECTED SIMULATION RESULTS:

             

Fig. 3. Steady-state responses at 50Hz without APF: (a) grid voltage v_g and grid current i_g, (b) harmonic spectrum of v_g, (c) harmonic spectrum of i_g.

Fig. 5. Steady-state responses at 50Hz with CRC controlled APF: (a) grid voltage v_g and grid current i_g, (b) harmonic spectrum of compensated i_g.

Fig. 4. Steady-state responses at 49.8Hz with CRC controlled APF: (a) grid voltage v_g and grid current i_g, (b) compensation current i_c, reference current i_ref and current tracking error, (c) harmonic spectrum of i_g.

Fig. 5. Steady-state responses at 49.8Hz with FORC controlled APF: (a) grid voltage v_g and grid current i_g, (b) compensation current i_c, reference current i_ref and current tracking error, (c) harmonic spectrum of i_g.

Fig. 6. Steady-state responses at 50.2Hz with CRC controlled APF: (a) grid voltage v_g and grid current i_g, (b) compensation current i_c, reference current i_ref and current tracking error, (c) harmonic spectrum of i_g.

Fig. 7. Steady-state responses at 50.2Hz with CRC controlled APF: (a) grid voltage v_g and grid current i_g, (b) compensation current i_c, reference current i_ref and current tracking error, (c) harmonic spectrum of i_g.

Fig. 8. Responses to step changes of grid frequency: (a) 49.5Hz→50.5Hz, (b)

50.5Hz→49.5Hz.

Fig. 9. Responses to step load changes at 49.8Hz fundamental frequency: (a) R 15Ω→30Ω, (b) R 30Ω→15Ω.

CONCLUSION:

This paper proposes a frequency adaptive FORC scheme with fixed sampling rate to track or eliminate any periodic signal with variable frequency. Using Lagrange interpolation based FD filter to approximate the fractional delay items in RC, the proposed FORC offers fast on-line tuning of the fractional delay and the fast update of the coefficients. It provides APFs with a simple but very accurate real-time frequency adaptive control solution to harmonics distortions compensation under grid frequency variations. The stability criteria of FORC systems are given, which are compatible with those of CRC systems. A study case of FORC based single-phase shunt APF is done. Experiment results show the effectiveness of the proposed FORC strategy. Furthermore, the Lagrange interpolation based FORC can be used in extensive applications, such as the feeding currents control of grid connected converters [10]-[11], [27], programmable AC power supply [28], active noise cancelation, and so on.

REFERENCES:

[1] H. Akagi, “New trends in active filters for power conditioning,” IEEE Trans. Ind. Applicat., vol. 32, no. 6, pp. 1312-1322, Nov./Dec. 1996.

[2] H. Akagi, “Active harmonic filters,” Proceedings of the IEEE, vol. 93, no. 12, pp. 2128-2141, Dec. 2005.

[3] Y. Han, L. Xu, M. M. Khan, C. Chen, G. Yao, and L. Zhou, “Robust deadbeat control scheme for a hybrid APF with resetting filter and ADALINE-based harmonic estimation algorithm,” IEEE Trans. Ind. Electron., vol. 58, no. 9, pp. 3893-3904, Sep. 2011.

[4] M. Angulo, D. A. Ruiz-Caballero, J. Lago, M. L. Heldwein, and S. A. Mussa, “Active power filter control strategy with implicit closed-loop current control and resonant controller,” IEEE Trans. Ind. Electron., vol. 60, no. 7, pp. 2721-2730, Jul. 2013.

[5] P. Mattavelli and F. P. Marafao, “Repetitive-based control for selective harmonic compensation in active power filters,” IEEE Trans. Ind. Electron., vol. 51, no. 5, pp. 1018-1024, Oct. 2004.

Application of Artificial Neural Networks for Shunt Active Power Filter Control

ABSTRACT:

Artificial neural network (ANN) is becoming an attractive estimation and regression technique in many control applications due to its parallel computing nature and high learning capability. There has been a lot of effort in employing the ANN in shunt active power filter (APF) control applications. Adaptive Linear Neuron (ADALINE) and feed-forward multilayer neural network (MNN) are the most commonly used ANN techniques to extract fundamental and/or harmonic components present in the nonlinear currents. This paper aims to provide an in-depth understanding on realizing ADALINE and feed-forward MNN-based control algorithms for shunt APF. A step-by-step procedure to implement these ANN-based techniques in MATLAB/Simulink environment is provided. Furthermore, a detailed analysis on the performance, limitation, and advantages of both methods is presented in the paper. The study is supported by conducting both simulation and experimental validations.

KEYWORDS:

1.      Adaptive Linear Neuron (ADALINE)

2.       Artificial neural network (ANN)

3.       Feed-forward multilayer neural network (MNN)

4.       Shunt active power filter (APF)

SOFTWARE: MATLAB/SIMULINK

CIRCUIT DIAGRAM:

Fig. 1. Shunt APF system configuration.

CONTROL SYSTEM:

Fig. 2. ADALINE used to extract the fundamental active load current amplitude.

Fig. 3. Shunt APF control template using either MNN or ADALINE structures

SIMULATION RESULTS:

Fig. 4. Dynamic performance of the feed-forward MNN shunt APF for a trained

load scenario.

Fig. 5. Dynamic performance of the feed-forwardMNNshunt APF for untrained load scenario.

Fig. 6. Dynamic performance of the ADALINE shunt APF.

CONCLUSION:

In this paper, two widely used ANN-based shunt APF control strategies are investigated: 1) the ADALINE; and 2) the feed forward MNN. A simple step-by-step procedure is provided to implement each method in MATLAB/Simulink environment. The ADALINE is trained online by the LMS algorithm, while the MNN is trained offline using the SCG back propagation algorithm to extract the fundamental load active current magnitude. The performance of these ANN-based shunt APF controllers is evaluated through detailed simulation and experimental studies. Based on the study conducted in this paper, it is observed that the ADALINE-based control technique performs better than the feed-forward MNN. For untrained load scenario, the feed forward MNN fails to extract the fundamental component, resulting in overcompensation from the dc-link PI regulator. On contrary, the online adaptiveness of ADALINE makes it applicable to any load condition.

REFERENCES

[1] P. Kanjiya, V. Khadkikar, and H. H. Zeineldin, “A noniterative optimized algorithm for shunt active power filter under distorted and unbalanced supply voltages,” IEEE Trans. Ind. Electron., vol. 60, no. 12, pp.5376–5390, Dec. 2013.

[2] B. Singh, K. Al-Haddad, and A. Chandra, “A review of active filters for power quality improvement,” IEEE Trans. Ind. Electron., vol. 46, no. 5, pp. 960–971, Oct. 1999.

[3] M. Popescu, A. Bitoleanu, and V. Suru, “A DSP-based implementation of the p–q theory in active power filtering under nonideal voltage conditions,” IEEE Trans. Ind. Informat., vol. 9, no. 2, pp. 880–889, May 2013.

[4] V. Silva, J. G. Pinto, J. Cabral, J. L. Afonso, and A. Tavares, “Real time digital control system for a single-phase shunt active power filter,” in Proc. Conf. Rec. INDIN, 2012, pp. 869–874.

[5] A. Hamadi, S. Rahmani, and K. Al-Haddad, “Digital control of a shunt hybrid power filter adopting a nonlinear control approach,” IEEE Trans. Ind. Informat., vol. 9, no. 4, pp. 2092–2104, Nov. 2013.